Table Of Contents
What is Mean Formula?
Mean refers to the mathematical average calculated for a set of two or more values. There are primarily two ways of calculating it: arithmetic means, where all the numbers are added and then divided by the number of items, and geometric mean, where we multiply the numbers together and then take the Nth root and subtract it with one.

The arithmetic mean formula determines the average value in the data. It is a vital function in education, healthcare, and the business world as it gives the individual an idea of the center value of the data set. In fact, it proves to be the yardstick for observations beyond the basic analysis or any data.
Key Takeaways
- Mean means the mathematical average estimated for two or more values.
- One may calculate it in two ways: arithmetic means, where all the numbers are added and then divided by the number of items, and geometric mean,
- where we multiply the numbers and then take the Nth root and deduct it with one.
- The arithmetic mean formula means calculating by adding all the available periodic returns and dividing the result by the periods' number.
- The geometric mean formula means calculating by initially adding one to each available periodic return, multiplying them, raising the result to the reciprocal power of the periods, and subtracting one from it.
Formula
The mean of a data set can be calculated through either geometric or arithmetic mean formula. Both formulae have been explained below.
The arithmetic mean formula is calculated by adding all the available periodic returns and dividing the result by the number of periods.
where Ri = return in the ith year and n = Number of periods
The geometric mean formula is calculated by initially adding one to each of the available periodic returns, then multiplying them and raising the result to the power of the reciprocal of the number of periods, and then deducting one from it.
Calculation
Now that we have understood the mean formula, let us see how the calculation process for it is executed through the discussion below.
Steps to Calculate Arithmetic Mean
- Step 1: Firstly, determine the returns for various periods based on the portfolio's value or investment at various times. The returns are denoted by r1, r2, ….., rn corresponding to 1st year, 2nd year,…., nth year.
- Step 2: Next, determine the number of periods denoted by n.
- Step 3: Finally, the arithmetic average of returns is calculated by adding all the periodic returns and dividing the result by the number of periods, as shown above.
Steps to Calculate Geometric Mean
- Step 1: First, determine the various periodic returns denoted by r1, r2, ….., rn corresponding to 1st year, 2nd year,…., nth year.
- Step 2: Next, determine the number of periods denoted by n.
- Step 3: Finally, the geometric average of returns is calculated by initially adding one to each of the available periodic returns, then multiplying them and raising the result to the power of the reciprocal of the number of periods, and then deducting one from it as shown above.
Examples
Let us understand the mean formula statistics through the detailed calculation and examples below.
Let us take an example of company stock with the following stock price at the end of each financial year.

Calculate the arithmetic and geometric mean of the annual returns based on the given information.
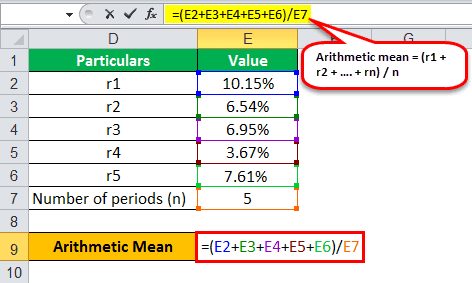
Return of 1st year, r1

- Return of 1st year, r1 = * 100%
- = * 100%
- = 10.15%
Similarly, we have calculated the returns for the year as follows:
Return of 2nd year, r2 = * 100%
= 6.54%
Return of 3rd year, r3 = * 100%
= 6.95%
Return of 4th year, r4 = * 100%
= 3.67%
Return of 5th year, r5 = * 100%
= 7.61%
Therefore, the calculation of the arithmetic mean equation is as follows:

- Arithmetic mean = (r1 + r2 + r3 + r4 + r5) / n
- = (10.15% + 6.54% + 6.95% + 3.67% + 7.61%) / 5
The Arithmetic Average of Returns will be:

Now, the calculation of geometric average equation is done as follows:

- Geometric mean = 1/n – 1
- = 1/5 – 1
The Geometric Average of Returns will be:

Therefore, the arithmetic and the geometric mean of the returns are 6.98% and 6.96% respectively.
Relevance and Uses
From the perspective of an analyst, an investor, or any other financial user, it is very important to understand the concept of mean formula statistics as it is a statistical indicator used to estimate a company's stock performance over a certain period, which can be days, months, or years.
Mean Formula In Excel
Now, let us take the example of the stock prices of Apple Inc. for 20 days to illustrate the concept of mean in the Excel template below.
The calculation of the arithmetic mean formula is as follows:


The geometric mean is as follows:


The table provides a detailed calculation of the arithmetic and geometric mean.